
L'esperimento dei ricercatori prevedeva pareti trasparenti per consentire il pieno accesso visivo e utilizzava una visualizzazione del flusso all'avanguardia. Credito:Foto:Michael Schatz
La turbolenza gioca un ruolo chiave nella nostra vita quotidiana, rendendo i viaggi in aereo accidentati, influenzando il tempo e il clima, limitando l'efficienza del carburante delle auto che guidiamo e incidendo sulle tecnologie per l'energia pulita. Tuttavia, scienziati e ingegneri si sono enigmi sui modi per prevedere e alterare i flussi di fluidi turbolenti, ed è rimasto a lungo uno dei problemi più impegnativi nella scienza e nell'ingegneria.
Ora, i fisici del Georgia Institute of Technology hanno dimostrato, numericamente e sperimentalmente, che la turbolenza può essere compresa e quantificata con l'aiuto di un insieme relativamente piccolo di soluzioni speciali alle equazioni che governano la dinamica dei fluidi che possono essere precalcolate per una particolare geometria, una volta per tutte.
"Per quasi un secolo, la turbolenza è stata descritta statisticamente come un processo casuale", ha affermato Roman Grigoriev. "I nostri risultati forniscono la prima illustrazione sperimentale che, su scale di tempo adeguatamente brevi, la dinamica della turbolenza è deterministica e la collega alle equazioni deterministiche sottostanti."
I risultati sono stati pubblicati in Proceedings of the National Academy of Sciences il 19 agosto 2022. Il team di ricercatori era guidato da Grigoriev e Michael Schatz, professori della School of Physics della Georgia Tech che hanno collaborato a vari progetti di ricerca negli ultimi due decenni.
Schatz e Grigoriev sono stati raggiunti nello studio dagli studenti laureati della School of Physics Chris Crowley, Joshua Pughe-Sanford e Wesley Toler, insieme a Michael Krygier, uno scienziato post-dottorato presso i Sandia National Laboratories, che ha sviluppato i risolutori numerici dello studio come studente laureato presso Georgia Tech.
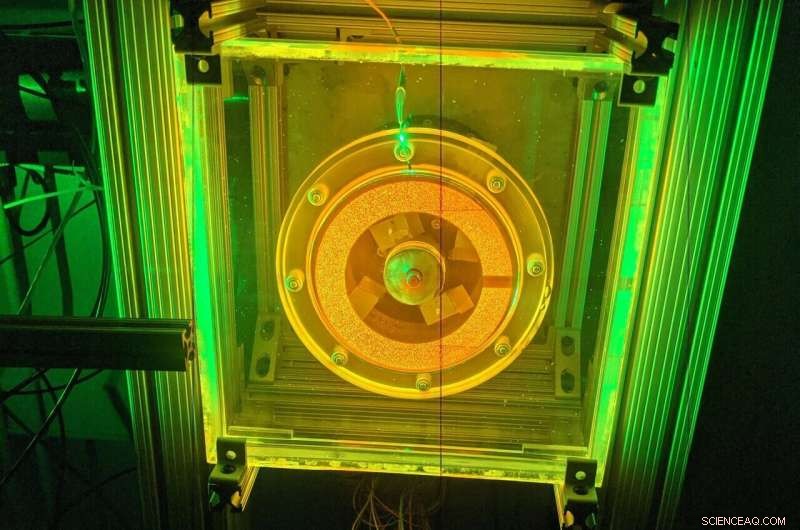
La configurazione ha consentito ai ricercatori di ricostruire il flusso tracciando il movimento di milioni di particelle fluorescenti sospese. Credito:Foto:Michael Schatz
Una nuova "tabella di marcia" per la ricerca sulla turbolenza
La previsione quantitativa dell'evoluzione dei flussi turbolenti - e, in effetti, di quasi tutte le loro proprietà - è piuttosto difficile. "La simulazione numerica è l'unico approccio predittivo affidabile esistente", ha affermato Grigoriev. "Ma può essere terribilmente costoso. L'obiettivo della nostra ricerca era rendere la previsione meno costosa."
I ricercatori hanno creato una nuova "tabella di marcia" della turbolenza osservando un flusso turbolento debole che era confinato tra due cilindri a rotazione indipendente, offrendo al team un modo unico per confrontare le osservazioni sperimentali con i flussi calcolati numericamente, a causa dell'assenza di "effetti finali" che sono presenti in geometrie più familiari, come il flusso lungo un tubo.
"La turbolenza può essere pensata come un'auto che segue una sequenza di strade", ha affermato Grigoriev. "Forse un'analogia ancora migliore è un treno, che non solo segue una ferrovia a un orario prestabilito, ma ha anche la stessa forma della ferrovia che sta seguendo."
L'esperimento prevedeva pareti trasparenti per consentire il pieno accesso visivo e utilizzava una visualizzazione del flusso all'avanguardia per consentire ai ricercatori di ricostruire il flusso tracciando il movimento di milioni di particelle fluorescenti sospese. In parallel, advanced numerical methods were used to compute recurrent solutions of the partial differential equation (Navier-Stokes equation), governing fluid flows under conditions exactly matching experiment.
It is well-known that turbulent fluid flows exhibit a repertoire of patterns—referred to as "coherent structures" in the field—that have a well-defined spatial profile but appear and disappear in an apparently random manner. By analyzing their experimental and numerical data, the researchers discovered that these flow patterns and their evolution resemble those described by the special solutions they computed. These special solutions are both recurrent and unstable, meaning they describe repeating flow patterns over short intervals of time. Turbulence tracks one such solution after another, which explains what patterns can appear, and in what order.
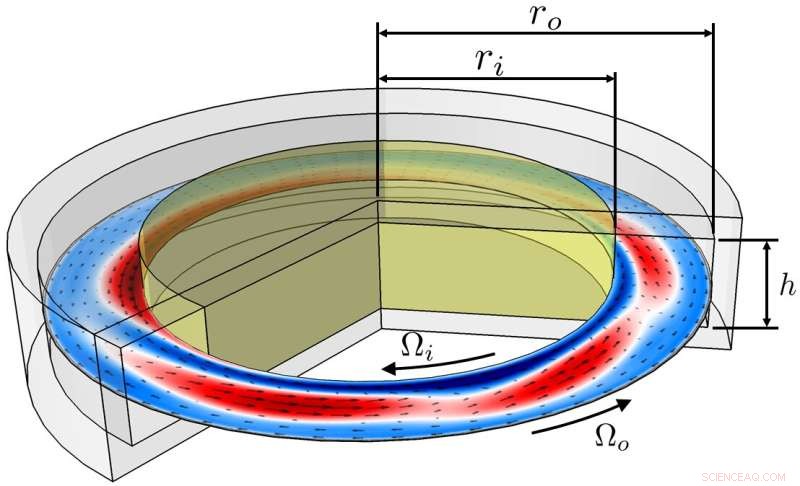
A schematic of the physicists' research. Credit:Michael Schatz, Roman Grigoriev.
Recurrent solutions, two frequencies
"All the recurrent solutions that we found in this geometry turned out to be quasi-periodic—that is, characterized by two different frequencies," said Grigoriev. One frequency described the overall rotation of the flow pattern around the axis of symmetry of the flow, while the other described the changes in the shape of the flow pattern in a reference frame co-rotating with the pattern. The corresponding flows repeat periodically in these co-rotating frames.
"We then compared turbulent flows in experiment and direct numerical simulations with these recurrent solutions and found turbulence to closely follow (track) one recurrent solution after another, for as long as turbulent flow persisted," Grigoriev said. "Such qualitative behaviors were predicted for low-dimensional chaotic systems, such as the famous Lorenz model, derived six decades ago as a greatly simplified model of the atmosphere."
The work represents the first experimental observation of chaotic motion tracking recurrent solutions actually observed in turbulent flows. "The dynamics of turbulent flows are, of course, far more complicated due to the quasi-periodic nature of recurrent solutions," Grigoriev added.
"Using this method, we conclusively showed that the organization of turbulence in both space and time is well captured by these structures," the researchers said. "These results lay the foundation for representing turbulence in terms of coherent structures and leveraging their persistence in time to overcome the devastating effects of chaos on our ability to predict, control, and engineer fluid flows."
A new dynamical foundation for 3D fluid flows
These findings most immediately impact the community of physicists, mathematicians, and engineers who are still trying to understand fluid turbulence, which remains "perhaps the greatest unsolved problem in all of science," Grigoriev said.
"This work builds and expands on previous work on fluid turbulence by the same group, some of which was reported at Georgia Tech in 2017," he added. "Unlike the work discussed in that publication, which focused on idealized two-dimensional fluid flows, present research addresses the practically important and more complicated three-dimensional flows."
Ultimately, the team's study lays a mathematical foundation for fluid turbulence which is dynamical, rather than statistical, in nature—and hence has the capability to make quantitative predictions, which are crucial for a variety of applications.
"It can give us the ability to dramatically improve the accuracy of weather forecasts and, most notably, enable prediction of extreme events such as hurricanes and tornadoes," said Grigoriev. "Dynamical framework is also essential for our ability to engineer flows with desired properties, for instance, reduced drag around vehicles to improve fuel efficiency, or enhanced mass transport to help remove more carbon dioxide from the atmosphere in the emerging direct air capture industry." + Esplora ulteriormente